CBSE CLASS 9TH SCIENCE(PHYSICS) CHAPTER MOTION (Graphical ) Part-2

CBSE CLASS 9TH SCIENCE(PHYSICS) CHAPTER MOTION (Graphical ) Part-2 

GRAPH:

A graph is a line, straight or curved which shows the variation of one quantity w.r.t other, which are interrelated with each other.

y=f(x)

In a relation of two quantities, the quantity which is made to alter at will, is called the independent variable.

The other quantity which varies as a result of this change is called the dependent variable. 

Graph

In any graph , the independent variable is represented along x-axis (Time). 

Dependent variable is represented along y-axis (Distance, Displacement, Speed , Velocity, Acceleration etc).

TYPES OF GRAPHS:

1. When object is at Rest

2. When Object is in Uniform Motion

3. When Object is in Non Uniform Motion (Increasingly and decreasingly )

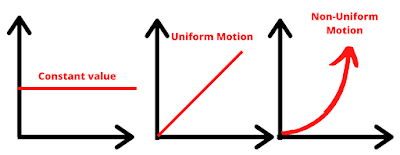Types of Graphs in Motion

Distance-Time Graphs: 

Distance time graph denoted by s-t graph shows the variation of distance covered by object taken on dependent axis with time independent axis. 
Slope of the distance-time graph represents speed.

1).Distance time graph for a stationary object:

The distance time graph for a object at rest is straight line parallel to time axis.

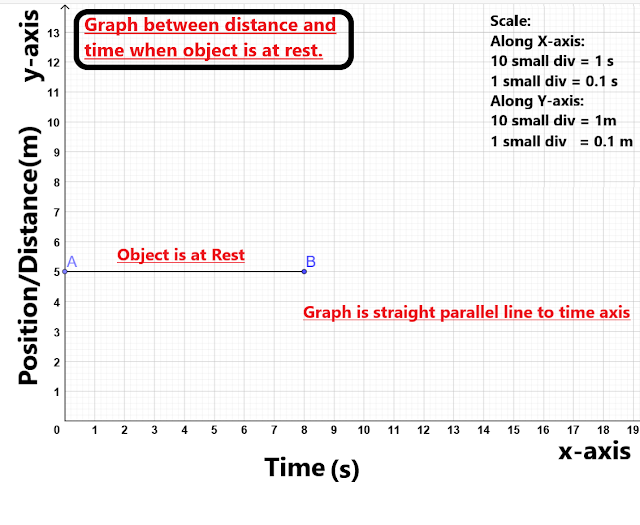Distance Time Graph When Object is at rest

2. Distance Time graph for Linear Uniform Motion:

For uniform motion along a straight line , the distance time graph is straight line inclined at angle with time axis.

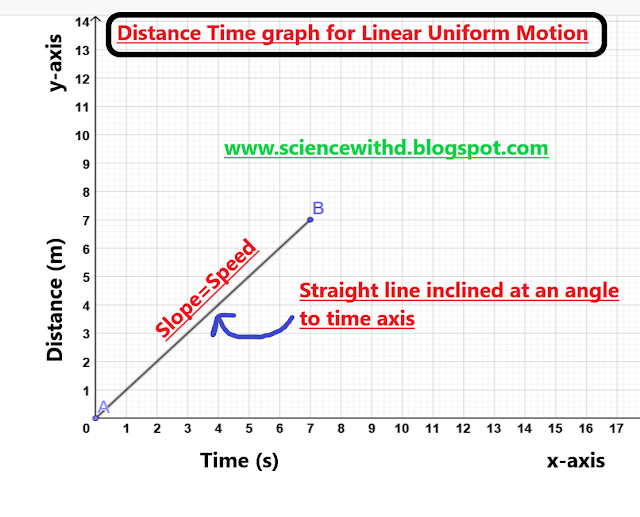Graph between Distance Time for Linear Uniform Motion

3. Distance Time graph for Non Uniform Motion:
For graph with increasing slope with time represents speeding up. 

The graph with decreasing slope represents slowing down.

4. Distance Time graph which are not possible:

Distance Time Graph which are not possible

DISPLACEMENT TIME GRAPHS:
Displacement time graph denoted by s-t graph shows the variation of displacement covered by object taken on dependent axis with time independent axis. 

Slope of the displacement-time graph represents velocity.

Displacement Time Graph

VELOCITY-TIME GRAPHS:

ACCELERATION-TIME GRAPHS:

SLOPE OF DISTANCE TIME GRAPH:

Slope of Distance time graph

Slope = Uniform speed = Distance travelled / time taken = Δx/Δt= x₂ – x_{1 /}t₂ – t₁  = (6-4)/ (6-4)= 1 m/s.

SLOPE OF VELOCITY TIME GRAPH:

Slope of velocity time graph

Slope = Uniform acceleration = velocity travelled / time taken = Δv/Δt= v₂ – v_{1 /}t₂ – t₁  = (6-4)/ (6-4)= 1 m/s².

AREA UNDER VELOCITY - TIME GRAPH:

1)AREA UNDER CONSTANT VELOCITY - TIME GRAPH:

DISTANCE COVERED BY OBJECT IS GIVEN BY AREA UNDER THE GRAPH AS SHOWN BELOW:

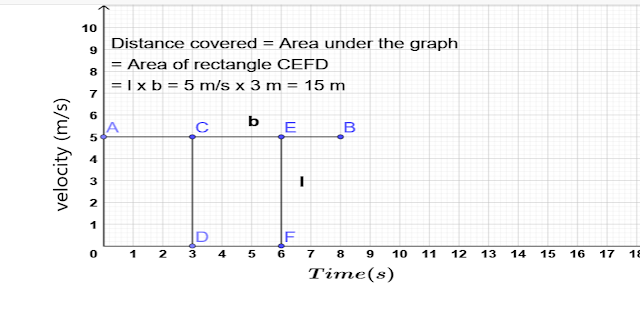
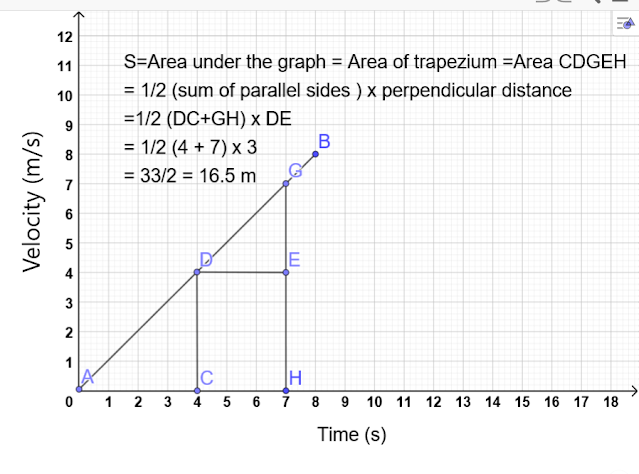
2) AREA UNDER LINEAR VELOCITY- TIME GRAPH :

DISTANCE COVERED BY OBJECT IS GIVEN BY AREA UNDER THE GRAPH AS SHOWN BELOW:

EQUATIONS OF MOTION:

For uniformly accelerated linear motion of an object velocity ,acceleration and the distance covered in a given time interval are correlated by set of three equations known as equations of motion.

1. VELOCITY - TIME EQUATION : v = u + at

2. POSITION - TIME EQUATION : S = ut + 1/2at².

3. POSITION - VELOCITY EQUATION : v² - u² = 2aS

1. DERIVATION OF VELOCITY - TIME EQUATION : v = u + at

Consider the velocity - time graph of an object that moves under uniform acceleration shown as

Slope = Acceleration (a) = BD/AD 

a = (BC-DC)/OC = (v-u)/t

a =(v-u)/t

at=(v-u)

v = u + at

2. POSITION - TIME EQUATION : S = ut + 1/2at².

Consider the velocity - time graph of an object that moves under uniform acceleration shown as

Velocity Time Graph

Distance covered by object = S = Area under graph

S = Area of trapezium OABDC 

S = 1/2 (sum of parallel side ) x perpendicular distance between parallel sides

S = 1/2 ( OA + BC ) x AD = 1/2 ( OA + BC ) x OC

S = 1/2 ( u + v ) x t          ( using v = u + at )

S = 1/2 ( u + u + at ) x t = 1/2 ( 2u + at ) x t

S = ( u + 1/2 at ) t = ut + 1/2at²

3. POSITION - VELOCITY EQUATION : v² - u² = 2aS

Consider the velocity - time graph of an object that moves under uniform acceleration shown as

Distance covered by object = S = Area under graph

S = Area of trapezium OABDC 

S = 1/2 (sum of parallel side ) x perpendicular distance between parallel sides

S = 1/2 ( OA + BC ) x AD = 1/2 ( OA + BC ) x OC

S = 1/2 ( u + v ) x t       (using  t = ( v - u )/a )

S = 1/2 ( u + v ) ( v - u )/a

2aS = (v + u ) ( v - u )

2aS = v² - u² 

v² - u² = 2aS